ggplot2 geomes for fmesher related objects

geom_fm is a generic function for generating geomes from various kinds of fmesher objects, e.g. fm_segm and fm_mesh_2d. The function invokes particular methods which depend on the class of the data argument. Requires the ggplot2 package.

Note: geom_fm is not yet a "proper" ggplot2 geom method; the interface may therefore change in the future.

Usage

geom_fm(mapping = NULL, data = NULL, ...)

# S3 method for class 'fm_mesh_2d'
geom_fm(
  mapping = NULL,
  data = NULL,
  ...,
  mappings = NULL,
  defs = NULL,
  crs = NULL,
  mapping_int = deprecated(),
  mapping_bnd = deprecated(),
  defs_int = deprecated(),
  defs_bnd = deprecated()
)

# S3 method for class 'fm_segm'
geom_fm(mapping = NULL, data = NULL, ..., crs = NULL)

# S3 method for class 'fm_mesh_1d'
geom_fm(
  mapping = NULL,
  data = NULL,
  ...,
  xlim = NULL,
  basis = TRUE,
  knots = TRUE,
  derivatives = FALSE,
  weights = NULL
)

Arguments

mapping: an object for which to generate a geom.
data: an object for which to generate a geom.
...: Arguments passed on to the geom method.
mappings: optional list of aes mappings for the non-triangle parts of the mesh, named "int" for interior constraint edges, "bnd" for boundary edges, and "loc" for the vertices.
defs: optional list of non-aes settings for the non-triangle parts of the mesh, named "int" for interior constraint edges, "bnd" for boundary edges, and "loc" for the vertices.
crs: Optional crs to transform the object to before plotting.
mapping_int, mapping_bnd, defs_int, defs_bnd: arguments; see mappings and defs.
xlim: numeric 2-vector; specifies the interval for which to compute functions. Default is data$interval
basis: logical; if TRUE (default), show the spline basis functions
knots: logical; if TRUE (default), show the spline knot locations
derivatives: logical; if TRUE (not default), draw first order derivatives instead of function values
weights: numeric vector; if provided, draw weighted basis functions and the resulting weighted sum.

Value

A combination of ggplot2 geoms.

Methods (by class)

geom_fm(fm_mesh_2d): Converts an fm_mesh_2d() object to sf with fm_as_sfc() and uses geom_sf to visualize the triangles and edges.

The mesh vertices are only plotted if mappings$loc or defs$loc is non-NULL, e.g. defs = list(loc = list()). Default argument settings:
```
... = linewidth = 0.25, color = "grey" # default for triangle mapping
defs = list(
  int = list(linewidth = 0.5, color = "blue"),
  bnd = list(linewidth = 1, color = "black"),
  loc = list(size = 1, color = "red")
)
```
geom_fm(fm_segm): Converts an fm_segm() object to sf with fm_as_sfc() and uses geom_sf to visualize it.
geom_fm(fm_mesh_1d): Evaluates and plots the basis functions defined by an fm_mesh_1d() object.

Examples

ggplot() +
  geom_fm(data = fmexample$mesh)

m <- fm_mesh_2d(
  cbind(10, 20),
  boundary = fm_extensions(cbind(10, 20), c(25, 65)),
  max.edge = c(4, 10),
  crs = fm_crs("+proj=longlat")
)
ggplot() +
  geom_fm(data = m)

ggplot() +
  geom_fm(data = m, defs = list(loc = list()))

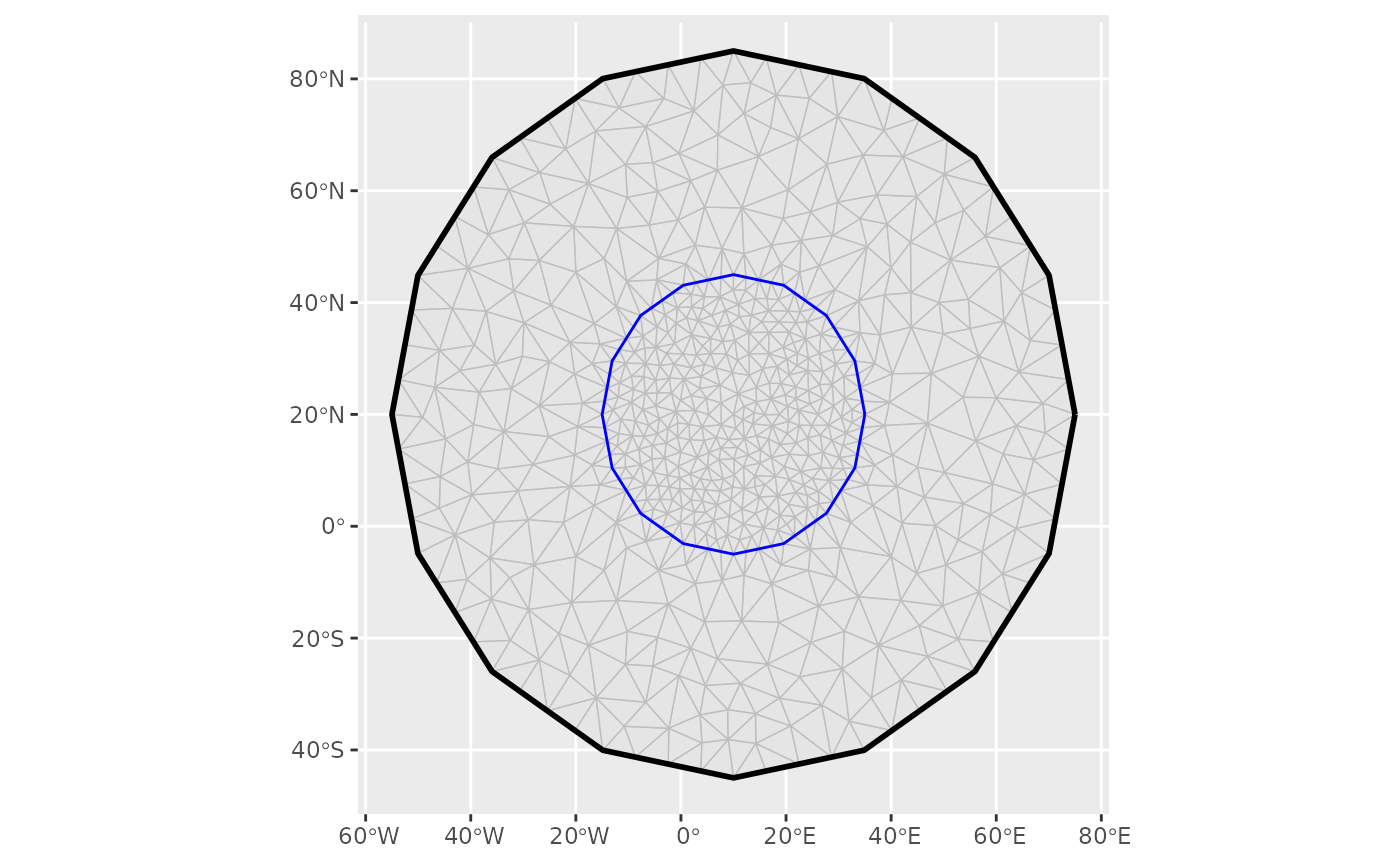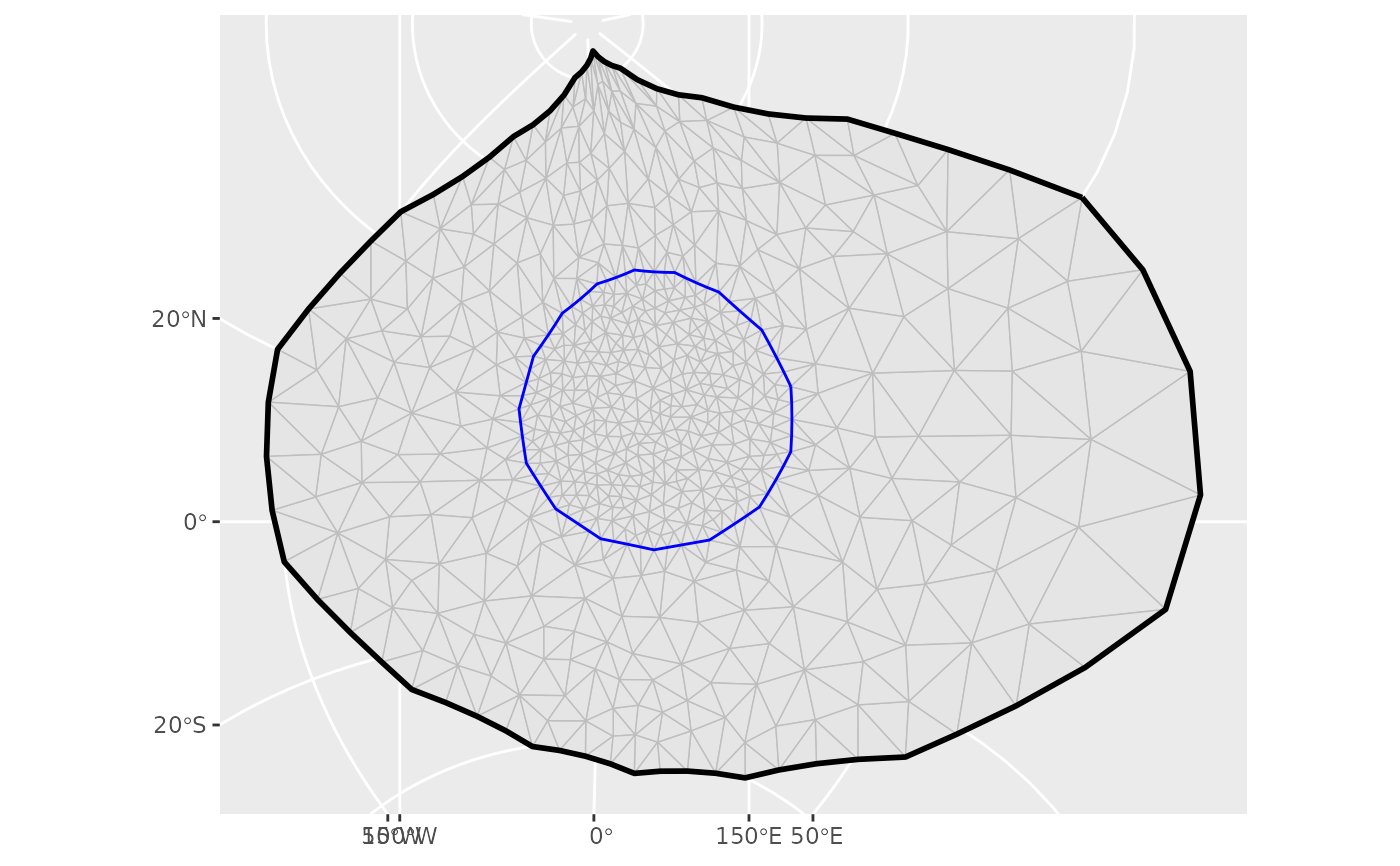ggplot() +
  geom_fm(data = m, crs = fm_crs("epsg:27700"))

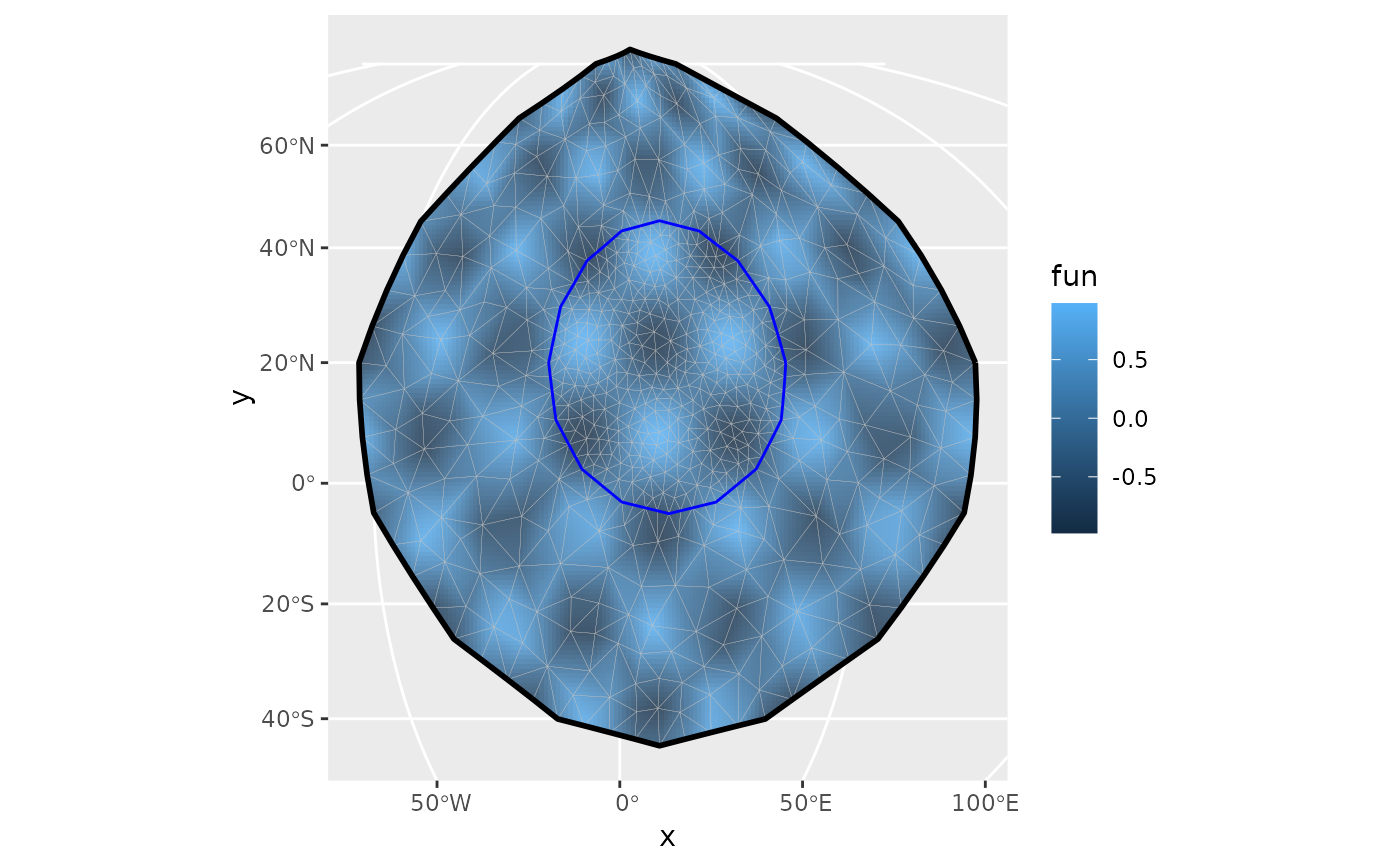# \donttest{
# Compute a mesh vertex based function on a different grid
px <- fm_pixels(fm_transform(m, fm_crs("mollweide_globe")))
px$fun <- fm_evaluate(m,
  loc = px,
  field = sin(m$loc[, 1] / 5) * sin(m$loc[, 2] / 5)
)
ggplot() +
  geom_tile(aes(geometry = geometry, fill = fun),
    data = px,
    stat = "sf_coordinates"
  ) +
  geom_fm(
    data = m, alpha = 0.2, linewidth = 0.05,
    crs = fm_crs("mollweide_globe")
  )

# }
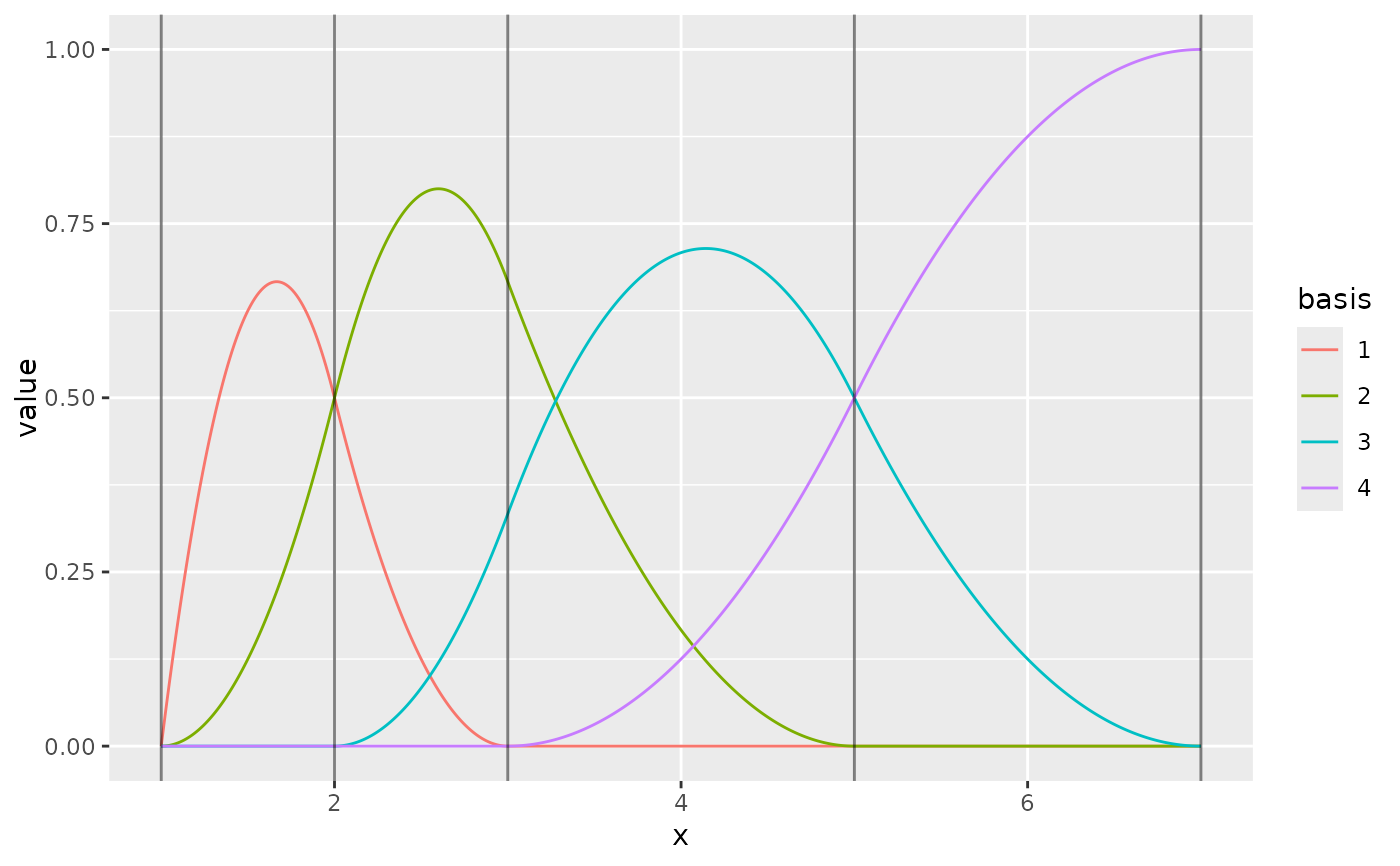
m <- fm_mesh_1d(c(1, 2, 4, 6, 10), boundary = c("n", "d"), degree = 2)
ggplot() +
  geom_fm(data = m, weights = c(4, 2, 4, -1))

m <- fm_mesh_1d(
  c(1, 2, 3, 5, 7),
  boundary = c("dirichlet", "neumann"),
  degree = 2
)
ggplot() +
  geom_fm(data = m)